Cell Division
The proposal that cells divide purely mechanically when they reach a critical size opens up fascinating perspectives. If cells bud off daughters in much the same way that drips bud from leaking taps, then division is something that just periodically happens to a cell. An extraordinary event overtakes it - the cell is torn in two.
If this is the case, then if both daughters of a dividing cell are to live, they must both have a full complement of the various internal components that are required for their self-maintenance. These components, in real biological cells, would be ribosomes, genes, mitochondria, etc. If a cell doesn't have a full comlement of components, it will most likely be unable to maintain itself, and will disintegrate.
Since a cell is going to divide in two, it has to have at least 2 copies of each component ready before division occurs, if there is to be any chance of both daughters having at least one of each component.
But, if the chance of any single component ending up in one daughter rather than the other is one in two, or 1/2, and there several different types of component, then the more components there are, the less likely it is that both daughters will contain at least one representative of each component. It becomes more likely that, in many cases, both copies of a component will end up in one daughter, leaving the other daughter devoid of that component. If one daughter ends up with both copies of one component, and the other daughter ends up with both copies of another component, then assuming that these components are crucial for cell maintenance, both daughter cells will not survive. Cell division will result in the death of one or both daughters. Clearly, if cells which divide are going to carry on living, they have to find ways to ensure that both daughters have a full complement of components.
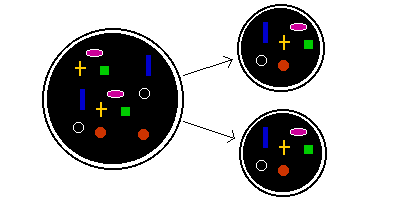
One way to ensure that both daughter cells get at least one copy of each cell component is to have multiple copies of each component. If there are 10 copies of each component, rather than just 2, it's unlikely that all 10 will end up in one daughter.
In fact, if there are n copies of a particular component, the probability of all n of them ending up in one daughter is 1/2n. And the probability that both daughters will have at least one copy of that particular component is thus 1 - 1/2n.
Given N different types of components, of which there are n copies of each, the probability P that both daughters will have at least one copy of each type of component is given by
P = (1 - 1/2n)N
|
Given this equation, it is possible to work out how many copies of each type of component are needed to be, say, 99% sure that both daughters will have at least one of each component. The table to the left gives the number of copies required for at least 99% probability of both daughters having one copy of each component. Thus, given only one component, there have to be 7 copies for >99% chance of both daughters getting a copy. But given a million components, there only need be 27 copies to achieve the same probability. |
Assuming that, as cells become more complex, they acquire more components, then assuming that each component has unit volume, the volume of components is N x n. As the numbers of components increase, the cell has to do a lot of work to produce the required numbers of copies. Also, as the number of components increases, cell size increases, threatening to trigger division.
One way that a cell can reduce the amount of work it has to do is to bundle components together. If a cell has 10,000 genes coding for different proteins, then if they are all separate, it needs to make 20 copies of each. But if the 10,000 genes are attached to each other in one giant chromosome, it only has to make 7 copies of each chromosome - assuming that there are no other components. The result is that the cell need do less than half the work, to produce fewer components.
But if each daughter cell only needs one copy of these chromosomes, it would be preferable if only 2 copies were made, rather than 7 copies. And there is a way of ensuring that both daughter cells each get one of the two copies. This relies on the fact that when a cell divides in two, the cell membrane divides into two parts. One half goes to one daughter, and the other half to the other, like a potato jacket being cut in half.
If one copy of a component (or set of components) is attached to the cell membrane at some point, and a second copy is attached to the cell membrane opposite, then when the cell divides, it's almost certain that one copy will be drawn into one daughter, and the other copy into the second daughter.
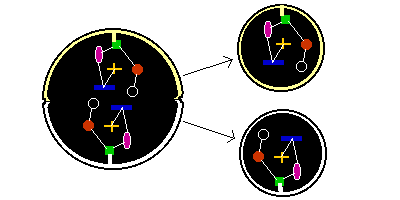
But how does a cell find the opposite internal face of the cell? If internal bracing struts appear within compressed cells, then one way to find opposite points would simply be to grow a rigid strut within the cell. If the ends of this strut can slip along the internal face of a spherical cell, then as the strut grows it will gradually reach a maximum length when the strut passes from one internal face through the centre of a spherical cell to the opposite face.
If the strut then continues to grow, and the cell walls resist, then the strut will bend. The bent strut will exert a force against the cell walls. If there are several struts, the net force exerted will be a simple multiple of the force exerted by a single bent strut. As the struts grow, they bend more, and the force they exert on the cell wall must tend to push the cell wall outwards, and draw the equatorial cell wall inwards.
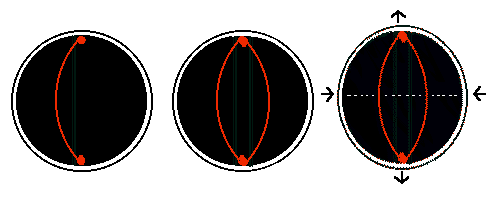
The point where bending stresses are highest is at the middle of the strut, and it is here that a strut is likely to break. When one strut breaks, the stress in the remaining struts will increase, and the likelihood is that they also will snap along the cell equator. It simply needs one copy of a chromosome to be attached to each end of these struts to ensure that a copy goes to each daughter.
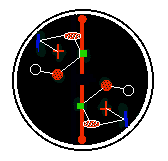
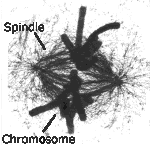And biological (eukaryote) cells do in fact do something like this, forming
spindles from one side of the cell to the other, on which the two
copies of the cell chromosomes are held. Other cell components, such a
ribosomes and mitochondria appear to exist in sufficient numbers in
the cell cytoplasm for them to most probably be represented in
both daughters after division.
This account of cell division all but inverts the standard DNA-centred explanation of cell division. Cell division happens because of the mechanical characteristics of the enlarging cell, and all other cell processes are a consequence of this division - multiple components, chromosome and other organelle formation, and spindle cells. Division is the primary event, and the cell components just go along with it, and have learned to live with it, and perhaps subsequently to acquire some degree of control over it.
If there is any truth in this mechanical account of cell division, then there is no more a "goal" to reproduction than it is the "goal" of a tap to produce drips. Reproduction - cell division (and this includes both mitosis and meiosis) - just happens spontaneously, in the right circumstances. Life isn't trying to reproduce. Cells just happen to divide occasionally. They would do so even if they were entirely devoid of genes. The role of genes is to provide instructions for synthesizing proteins, as one part of the many processes that tke place within cells.
In this approach, division was one of the first developments in the evolution of cells. And assuming that the earliest cells contained multiple copies of a few simple components, cell growth regularly resulted in division with both daughters containing a full complement of components. If then, over time, new components were added, then as the numbers of different components increased, the likelihood of successful reproduction decreased. Cells then began increasing the likelihood of success by reducing the numbers of components, by bundling them together into larger units - organelles. When the number and size of components grew still further, cells began to form spindles to hold components to opposite internal faces of the cell, and reduced the number of copies of each component to just two.